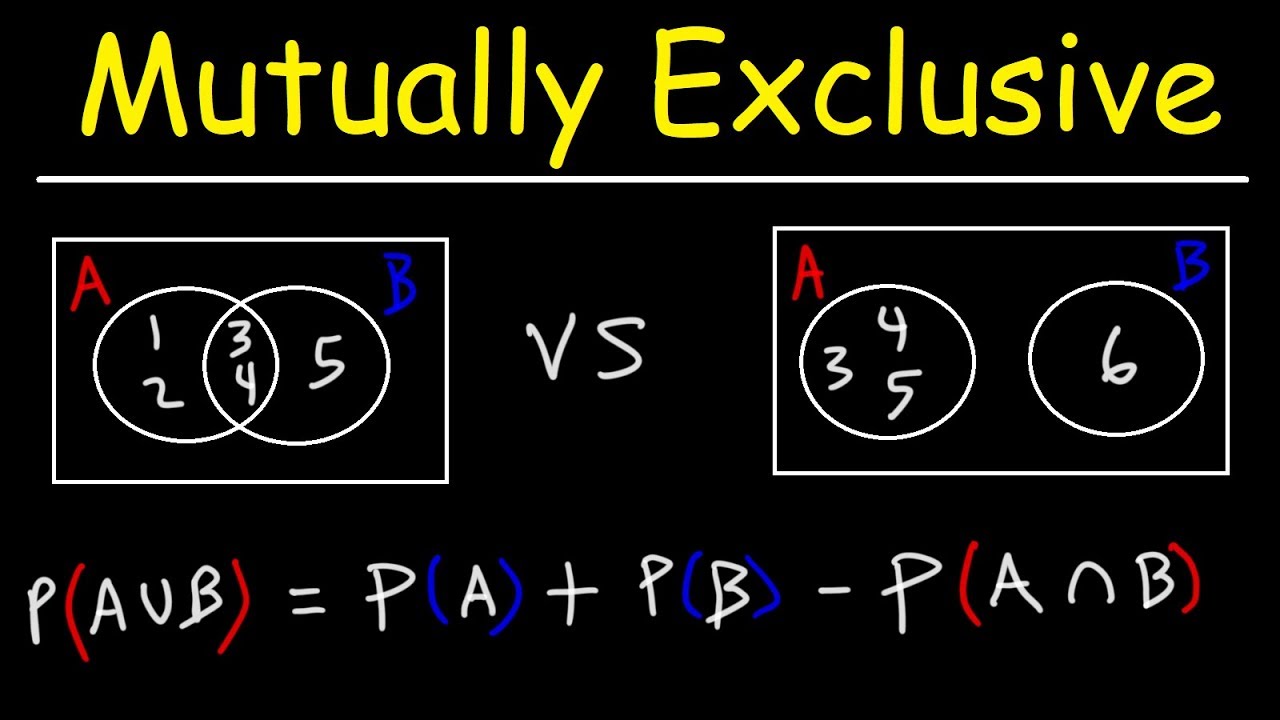Non Mutually Exclusive Venn Diagram

Venn diagrams and mutually exclusive.

Non mutually exclusive venn diagram. The first 6 worksheets each contain worked examples related questions and worked solutions. Take out a standard deck of 52. If the union of two mutually exclusive sets is the universal set they are called complementary. This booklet contains 6 gcse maths worksheets.

Turning left and turning right are mutually exclusive you can t do both at the same time. P english 14 40 7 20 total number of students taking english out of the total number of students. The following venn diagram represents mutually exclusive disjoint sets. The two events are such that e1 e2 φ the two sets e1 and e2 have no elements in common and their intersection is an empty set since they cannot occur at the same time.

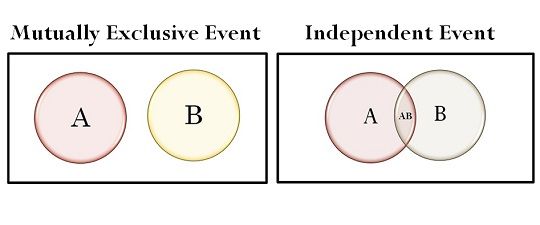
This probability video tutorial provides a basic introduction into mutually exclusive events with the use of venn diagrams. In the venn diagram above the probabilities of events a and b are represented by two intersecting sets i e they have some elements in common. In each venn diagram above the sample space of the experiment is represented. In a venn diagram the sets do not overlap each other in the case of mutually exclusive events while if we talk about independent events the sets overlap.

We saw that a mutually exclusive event is the name given to events that cannot happen if another event occurs while a non mutually exclusive event is an event that can happen no matter what. Statistics topics include cumulative frequency curves simple probability mutually exclusive events venn diagrams non mutually exclusive events venn diagrams and independent events tree diagrams. The intersection of two complementary sets is the null set and the union is the universal set as the following venn diagram suggests. In a venn diagram with 2 circles there are 6 main parts to consider.

Two events are non mutually exclusive if they have one or more outcomes in common. Can t happen at the same time. Use the venn diagram on the right to explain how the answer to the probability question was derived. Let s take this basic knowledge or sets and venn diagrams a bit further to understand how they relate to mutually exclusive and non mutually exclusive events.

Two mutually exclusive events two events are mutually exclusive if they cannot occur at the same time.